 |
|||
|
|
|||
|
Page Title:
Physical Properties |
|
||
| ||||||||||
|
|  DOE-HDBK-1129-99
3
Moles of T
and
He Versus Time, Per Mole of Tritium At the Start, In A Container Starting With Pure
2
Tritium At t=0
2.00
Mo
les
1.80
Per
mHe3At-t
Mo
le
mT2At-t
1.60
Of
Trit
mTotAt-t
1.40
iu
m
At
1.20
t=0
m=moles
1.00
x {l[t 0.5]/12.323}
n
=
m
e
m (T2 at tyears)
(years)
(T2 initial)
{1 - e {[t
}
x ln 0.5]/12.323}
m (He3 at t years)
=2m
(years)
0.80
(T2 initial)
{2 - e {[t
}
x ln 0.5]/12.323}
m (T2 + He3at tyears)
=
m
(years)
(T2 initial)
0.60
0.40
0.20
12.
15.
18.
21.
24.
27.
30.
33.
36.
40.
43.
46.
49.
52.
55.
58.
61.
64.
67.
70.
73.
0.00 0.0
3.0
6.1
9.2
32
40
48
56
64
72
80
88
96
05
13
21
29
37
45
53
61
69
77
85
93
00
81
62
42
3
4
5
5
6
7
8
8
9
0
1
1
2
3
4
4
5
6
7
7
8
Elapsed Time In Years In Increments Of 1/4 Half Life
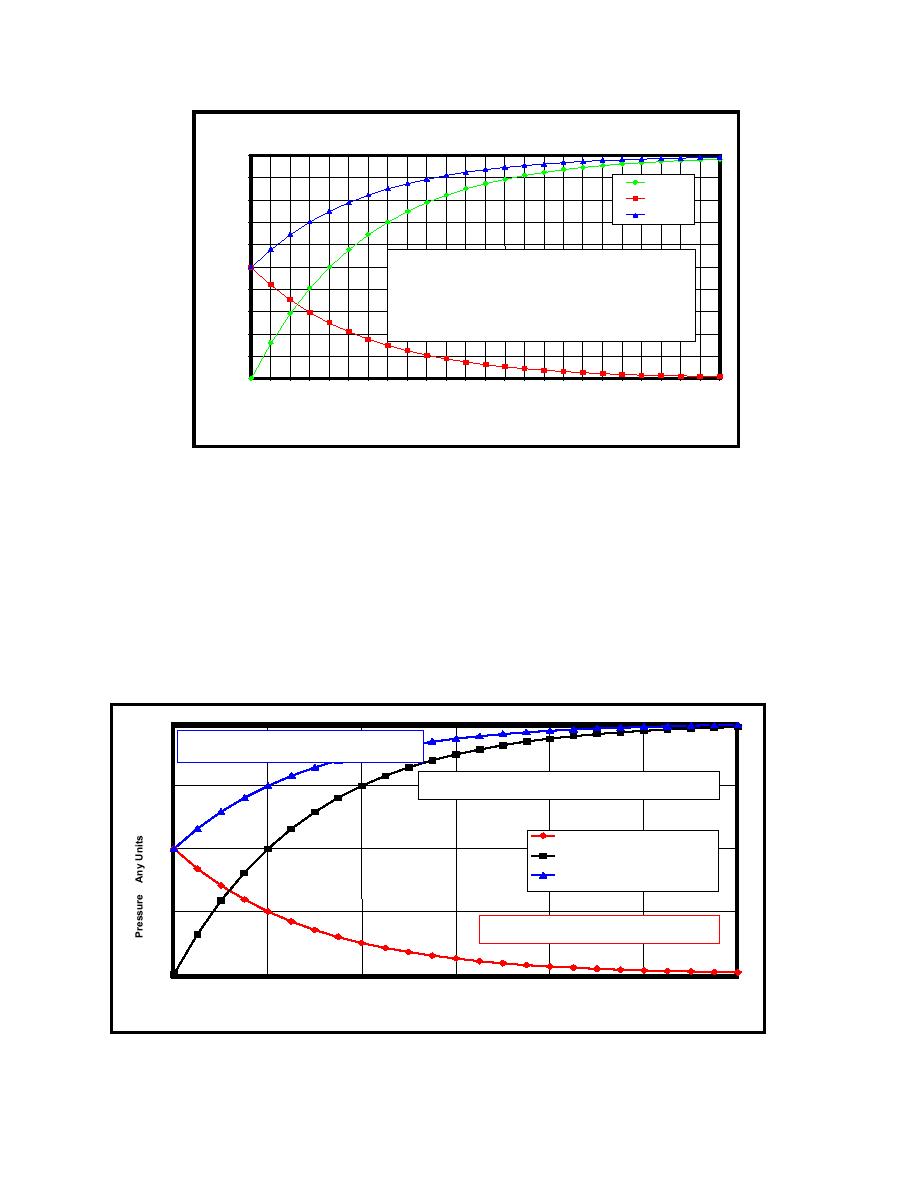
FIGURE 2-1. Rate of tritium decay of one mole of tritium
2.2 Physical Properties
Tritium gas is colorless, odorless, tasteless, and radioactive. It decays to 3He, a monatomic gas,
by emitting an electron and neutrino from the nucleus. Tritium has a high coefficient of diffusion. It
readily diffuses through porous substances such as rubber and can also diffuse through metals.
As tritium decays in ca container of constant volume at a constant temperature, the tritium partial
pressure decreases and the partial pressure of 3He increases. The pressure in the container
approaches twice that of the original container pressure. The rate of pressure change over time is
shown in Figure 2-2.
2.0
- e{[t (years) x ln 0.5]/12.323} )
P(Total at
t years) =P(T2 initial) (2
P(He3 at t years) =2P (T2 initial) (1-P(T2 initial) e {[t (years) x ln 0.5]/12.323} )
1.5
T2 Partial Pressure
1.0
He3 Partial Pressure
T2 Partial Pressure + He3 Partial
Pressure
0.5
e {[t(years)
x ln 0.5]/12.323}
P(T2 at
=P(T2
t years)
initial)
0.0
0.000
12.323
24.646
36.969
49.292
61.615
73.938
Time in Years
Time Period Shown = 6 Half-Lifes
FIGURE 2-2. Pressure versus time in a container of tritium
4
|
|
Privacy Statement - Press Release - Copyright Information. - Contact Us |
Click
here for thousands of PDF manuals